How to use comparison bar models in your classroom

Do you find yourself constantly watching your class struggle with problem-solving? Try using a bar model. This deceptively simple maths mastery technique might be the key to developing a deep and sustainable understanding of maths.

Comparison bar models are a type of bar modelling. You can apply them across the curriculum for any problem where learners need to compare two or more amounts.

Let’s look at how comparison bar models work and explore ideas for using them in your classroom.

What is a comparison bar model?

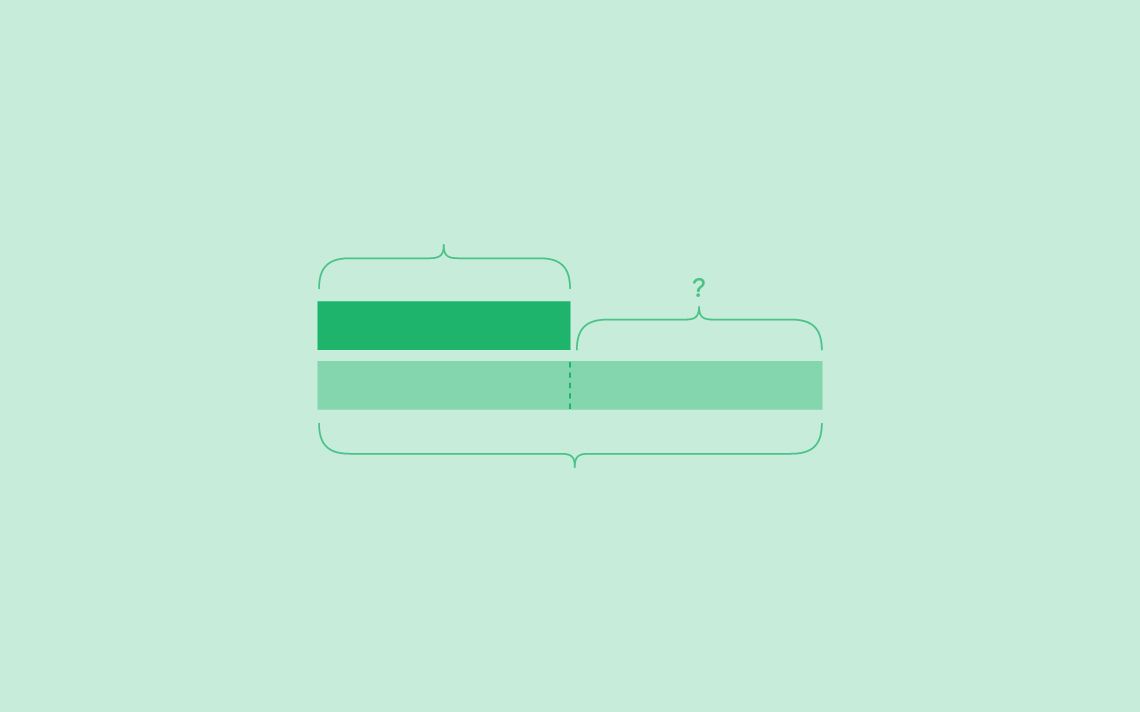
A comparison bar model uses solid bars to show known and unknown amounts. Bars are arranged vertically underneath each other so learners can see the difference in the length of the bars. The brackets show the total.

Here’s a question from Maths — No Problem! Textbook 3B, Lesson 13, along with its comparison bar model.

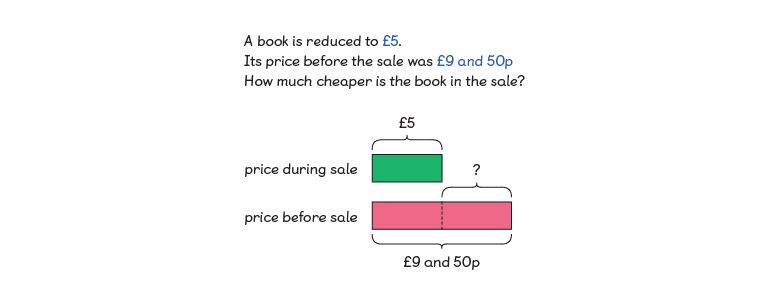
The great thing about comparison bar models is that they’re fun, approachable, and offer a simple starting point. Different colours and lengths of bars help learners see the difference between amounts. They’re also accessible — especially for struggling learners.

What are the benefits of using comparison bar models?

Comparison bar models are a simple way to show your learners what key mathematical vocabulary looks like. Putting it in a visual form rather than explaining an abstract concept supports learners’ conceptual understanding.

You might be familiar with using the acronym RUCSAC to help learners tackle word problems. RUCSAC stands for:

• Read the question
• Underline to find keywords
• Choose the operation(s) needed
• Solve the calculation
• Answer the question
• Check to see if the answer is correct

RUSAC gives learners a step-by-step method to solve problems. However, learners can get stuck on the ‘Underline to find keywords’ part which makes it hard to choose the most appropriate operation. It makes sense that teachers would turn to bar modelling as an effective replacement for RUCSAC.

Using comparison bar models across the curriculum

Comparison bar models work when there is a difference between amounts. You can apply them to different areas of maths, including money, time, and measurement.

Often you see vocabulary like:

• More than
• Less than/fewer than
• Heavier than
• Taller than
• Larger than
• Smaller than
• As many as
• As much as

Solving addition and subtraction problems

Comparison bar models are perfect for ‘how many more?’ and ‘how many fewer?’ questions. They tackle the misunderstanding that ‘fewer’ will always need subtraction and ‘more’ means add.

Introduce them to your class, start by talking about two different amounts.

Ask, “which has more, which has fewer?”

You can encourage learners to use different colours to draw their own bars on squared paper to represent the amounts. One bar will be shorter than the other. Then, it’s a simple step to ask how many more or fewer one bar has.

To help you get started, here are some example questions:

• I have 5 sweets. My sister has 4 more than me. How many does she have?
• I ran 7km on Saturday and 5km on Sunday. How many more kilometres did I run on Saturday? (In this question ‘more’ needs subtraction not addition)
• Billy has 25 marbles. Sally has 35 more than him. How many do they have in total?
• Alf, Bart, and Carina have some buttons. Alf and Bart have 185 buttons. Carina has 101 buttons. Alf and Bart give Carina the same number of buttons. They all now have the same amount. How many buttons did Alf give Carina?
• There were 24 fewer children than adults at a cinema. After ²/₅ of the children and ²/₃ of the adults left, there was the same number of children and adults at the cinema. How many people went to the cinema altogether?

As you can see, the questions grow in complexity. But bar models are there to help learners visualise the difference in amounts and find a starting point for calculating the solution.

Solving ratio problems

You don’t have to limit yourself to addition and subtraction. Comparison bar models can be great for ratio questions too:

• Ellie and Alisha have trading cards in the ratio of 2 to 3. They have 30 cards altogether. How many cards does Alisha have?
• I am making a necklace. I want 5 blue beads for every 3 yellow beads. If I’ve used 40 beads, how many were blue, and how many were yellow?
• I’m planting bulbs in my garden. I plant 3 daffodils for every 4 tulips. I’ve planted 60 daffodils. How many tulips have I planted?

Tackling the pitfalls of bar modelling

Bar modelling takes time to get right and like most teaching methods, it comes with pitfalls.

It’s worth taking some time to look at the questions you plan to use with your class. Practice them on your own first to avoid any confusion in the classroom.

Learner misconceptions

Look at examples of completed bar models with your class before they make their own. When they first start, you will find a range of misconceptions to address.

You might catch your learners:

• Not lining up each bar on the left-hand side
• Making spaces between the sections of a bar (rather than dividing it with lines)
• Choosing a comparative bar model when no comparison is needed
• Associating ‘more than’ to addition and ‘fewer than’ to subtraction

Teacher misconceptions

It can be easy to rely on comparison bar models so remember to use a range of representations in your classroom practice.

Bar modelling isn’t the end goal of teaching primary mathematics. Sure they are useful, but eventually, you want your learners to move on to abstract understanding. Think about progression and encourage them to move on when ready.

Comparison bar models are essential for a range of problem-solving questions. By now you should have a pretty clear picture of why they’re simple to use and don’t need any equipment. Plus, they come in handy when it’s time to record ideas in maths journals.

Bar models are so much more than a nifty trick for solving problems. The more you use them, the more intuitive it becomes for learners to visualise problems. If you want to make maths accessible and build your learners’ fluency skills, it might be time to give bar modelling a try in your classroom.