How to move from concrete resources to abstract learning

Children in my class find it easy to use concrete, practical resources in maths. I’ve found that they enjoy exploring new concepts in real-life situations. The tricky part comes when you try to move them on and apply this experience to solve abstract problems.

How the CPA approach fits into maths mastery

Concrete Pictorial Abstract (known as the CPA approach) is a key feature of the maths mastery approach. In a nutshell, the CPA approach uses concrete manipulatives to eventually gain a secure understanding of more abstract mathematical concepts.

What is CPA in maths mastery?

CPA (known as CRA in the US) is a research-based approach put forward by American psychologist Jerome Bruner in 1966. He proposed that representation in maths had three clear stages:

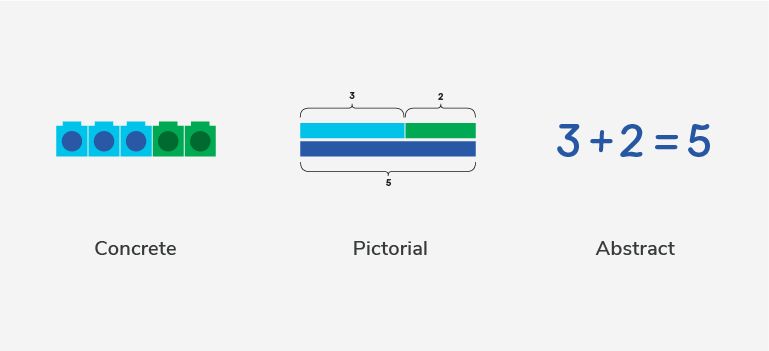
Stage 1: Concrete and action-based representation using manipulatives like money, counters, cubes, base ten blocks and dice to explore and solve problems — think of it as the ‘doing’ stage.

Stage 2: Pictorial representations of concrete objects such as bar models are used to model problems — the ‘seeing’ stage.

Stage 3: Abstract notations using mathematical symbols — the symbolic stage.

What’s the difference between concrete and abstract thinking?

At each stage of the CPA journey, learners build on their knowledge and deepen their understanding.

The concrete stage helps to bring concepts to life. As they move to the pictorial stage, the concrete stage helps learners make connections between the objects they just handled and pictorial representations. The final abstract stage stands on the shoulders of the concrete and pictorial exploration.

Let’s look at an example: to solve a multiplication problem, children must first understand what multiplication means.

Here are some ways to introduce the concept of multiplication:

• Making groups of counters, cubes, and other manipulatives
• Exploring words that mean the same as ‘multiply’
• Seeing and making pictures of cubes, blocks, and other objects in groups and arrays

Then, when they see the symbol for multiplication, they have a deeper understanding of the core idea.

How to move from concrete to abstract learning

Traditionally, using concrete resources in your teaching practice was limited to the early years’ classroom (with pressure to remove them as soon as possible).

The maths mastery approach is a bit different. Yes, children are expected to move on from manipulatives. But this doesn’t mean banning manipulatives from KS2 classrooms completely. Children grasp concepts at different rates, so they might move to abstract representations in some areas but remain at the concrete phase for others.

Here are three simple ways to move your learners from concrete to abstract thinking:

1. Move flexibly between CPA stages

CPA is usually linear, but it doesn’t have to be. You can move back to the concrete or pictorial representation stage when introducing new concepts, adding a challenge, or if students are not feeling secure about a topic.

Instead of rushing to give up manipulatives, make them available for all children to use. Encourage your class to see these resources as useful for everyone, rather than just for children at risk of being left behind or those with special educational needs. Varying the resources to represent concepts in different ways can help create connections between the concrete and abstract phases.

2. Use appropriate scaffolding

Think of scaffolding as breaking learning down into chunks and providing the tools a child needs to learn each chunk.

Scaffolding can include:

• Targeted questioning
• Sentence starters
• Example diagrams
• Modelling and discussing your thought process
• Key vocabulary cards
• Paired work
• Discussion time with a partner, group or whole class

CPA is a handy strategy, but it’s up to you to use it effectively. Scaffolding in mathematics is an art form. It’s knowing what to do just when a child needs your help. Too much scaffolding will create a passive learner, reliant on adult help. Too little scaffolding will prevent a child from making as much progress as they can in the lesson.

When you begin a new area of learning, you may need to provide lots of scaffolding. This should reduce as the children become more confident and develop their understanding.

3. Give slightly more challenging examples

When children appear to grasp a concept, it’s easy to rush them on to something new. Later, when you revisit the area of maths, why is it that they appear to have forgotten everything and you find yourself starting again at the beginning with manipulatives?

Instead of this frustrating process, spend more time making the link between CPA phases by incrementally offering slightly more challenging examples. This removes the rush to always be teaching the next thing and makes sure your class has good understanding.

Adding extra challenges could include:

• Presenting the same learning in a different context
• Increasing the complexity of the problem
• Adding in different stages to find the solution
• Asking children to create their own examples

Using concrete manipulatives is essential to secure understanding and fostering a rich mathematical experience. The concrete and pictorial stages are important parts of the learning process and should be valued, not rushed through.

Often, children need support to move from concrete to abstract thinking. By carefully structuring your move through CPA stages, you provide the support your children need, when they need it.