How do games in maths promote effective learning?

Complementing the work of Jean Piaget and Jerome Bruner, Dienes shifted mathematics teaching away from dry, abstract ideas and towards play in which the children themselves are actively involved and constructing learning from their experiences with a range of materials.

Dienes saw mathematics as a creative art. He believed maths should be a fun, explorative process that encourages children to think freely, and creatively. He noticed that when given toys or any kind of structured materials young children often felt the urge to classify them, then move on to ordering them and then transform them in some way. I saw this recently when a five year old showed me a range of dolls, some with haircuts and some without. Look around your classroom, do you see your pupils engage in similar play?

Dienes’ stages of learning theory

Seeing mathematics as “a gold mine for an indefinite supply of games”, Dienes went on to develop a six-stage theory for learning mathematics, with a focus on games. Many aspects of this theory are easy to identify in the Maths — No Problem! mastery approach through the anchor activities and stories and pictures in the textbooks. Dienes’ theory reflects Bruner’s Concrete Pictorial Abstract (CPA) stages. He advocates the use of manipulative materials, games and stories in maths, believing that children can learn more complicated maths at a younger age than had previously been thought.

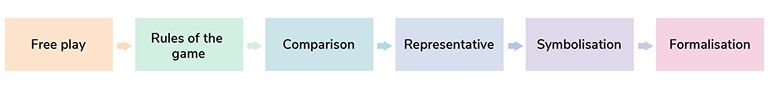
1. Free play

Dienes’ first stage uses games to enable initial trial and error and free exploration of materials. Here, learners familiarise themselves with the materials.

2. Rules of the game

This is followed by a stage where the regularities of the materials are identified and ‘rules’ are created. Once a number of similar games have been played in class, a discussion begins.

3. Comparison

Pupils compare the games, identifying similarities and differences between them. They articulate their discoveries before moving in the direction of abstraction instead of being completely absorbed in the concrete and physical playthings.

4. Representation

In the fourth stage, it’s useful to provide some kind of pictorial representation such as a chart, table, arrow diagram or coordinate system to anchor the patterns and abstractions in a visual way.

5. Symbolisation

Here, these ideas are mapped and pupils start to form a language around games.

6. Formalisation

Lastly, pupils begin to use what they’ve learned in the previous stages to test, prove and apply their own set of truths and theories around games.

Using games in the classroom

While we may not use this approach step by step in our daily lessons, we can understand what Dienes proposed and why.

We can seek to use those moments in lessons where it makes sense. We can have conversations about patterns that pupils spot, rules they begin to apply, symbols that depict abstractions usefully and the rich language around questions like “would it work if…?” or “what will happen if I try….”.

Dienes’ believed that games are an essential part of the foundation that mathematical concepts are built on. As the creator of the base 10 materials used widely in Key Stage 1 classrooms, he promoted the use of a variety of materials and experiences. He believed this would support embodied knowledge and situated cognition, instead of relying on learning organised around abstractions.

So, as you continue your maths lessons this week, where can you spot Dienes’ influence in your lessons? How have his theories been incorporated in the themes you are teaching? What range of materials will your pupils use in Maths lessons this week to root their learning in experience? How do you incorporate games in your Maths lessons? And what patterns or rules can you spot in the game you play on your phone, on the bus on your way to work? Because that’s Maths!