Understanding Relational and Instrumental mathematics

As a teacher, there are many moments where I find myself torn between finding the correct calculation method to follow, or just looking at the numbers and solving the problem by steps that appear logical to me. My question: is that just me? Or do you experience the same?

During my schooling, maths was a matter of following procedures and rules, and involved endless practice. I could follow and apply the steps as required, but understand little of it in a deeper, interconnected way.

During a recent Year 4 lesson, my class worked on an addition word problem as an anchor task. The class came up with a number of strategies to solve the problem including drawing number lines, using column addition, partitioning and recombining numbers. As individual pupils explained their thinking, peers could listen and make their own mathematical connections from what they heard. This is a totally different lesson approach from one I might have taught in the past: explaining one method to solve a problem, and letting pupils practice it repeatedly. The question is: which approach is better?

The Conflict of Approaches

Twentieth century mathematician turned psychology professor Richard Skemp’s concept of the conflict of approaches can come in handy here. Skemp identifies two primary approaches to maths: Instrumental and Relational. Instrumental mathematics centre around rote learning, memory, rules and correct answers. Relational mathematics focus more on establishing connections, building understanding over time, applying concepts to other problems, and gradual increases in complexity.

In reality, an effective maths lesson calls for a bit of each approach. However, an emphasis on the Relational approach leads pupils to a deeper understanding of maths, and a boosted confidence in the long run.

The Instrumental (or, procedural) method can sometimes be easier to pick up. It provides rules to get the right answers, and can be a rewarding option for arriving at the correct answer quickly. The risk? It doesn’t promote a deeper understanding of maths as found in the Relational (or, connected) approach — where what is learnt can be adapted to new tasks, becomes easier to remember, and exists on an intuitive, organic level.

When we begin a maths lesson with an anchor task, we are inviting students to solve a problem in their own way. This approach deliberately provides multiple entry points to knowledge or solutions, and each student can draw on their own prior learning and experiences to solve the problem. In doing so, they establish connections between concepts, and build upon their existing foundation of knowledge.

This Relational approach fosters an ethos of exploration, and develops confidence in ‘getting there’ — even when a pupil can’t yet see the big picture. Instrumental lesson design, however, can limit opportunities to build connections between mathematical concepts. Why? Because pupils are too often instructed to solve a problem in one way — and that’s it. Sure, they might know what to do — but not why they do it that way.

Your Teaching Journey

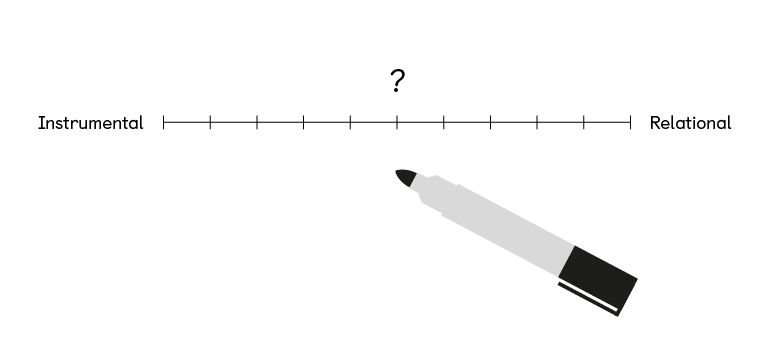
It’s useful to imagine or draw a line on a page with one end labelled ‘Instrumental Maths’ and the other labelled ‘Relational Maths.’ Mark where you think your maths teaching approach is and then, indicate a point further along the line towards which you are ready to ‘travel.’ Is there anything stopping you from making that journey? Decide on one or two practical steps to help you progress such as pairing with a colleague to develop an approach collaboratively.

Lastly, it seems to me that our biggest challenge in instructional design is making sure the pupils are doing the thinking, reasoning and connection-building during lessons, not teachers. Our maths lessons need to enable not just pupils’ technical abilities — but also a sense of creative thinking, self-discovery and exploration. The final question (and, it’s a big one!): wouldn’t that make maths a more positive, meaningful experience for every learner?