Dissecting a Year 6 maths word problem

Chapter 6 of the Maths — No Problem! Primary Series Workbook for Year 6 pupils includes a series of maths lessons in which pupils encounter complex maths word problems that can induce a cold sweat in the novice mathematician — pupil and staff-member alike.

Solving these problems demands higher-order thinking skills, fluency in the four mathematical operations, an aptitude for working with bar models and a whole new level of cooperation, discussion and reasoning.

Some of the more able learners will thrive on the challenge these rich, complex lessons offer. Even so, the depth of their understanding will be truly tested.

The following is the step-by-step solution that Sophia, a student at our school, used to solve one of these problems when she was in Year 6. The passages in quotation marks describe in her own words how she went about it.

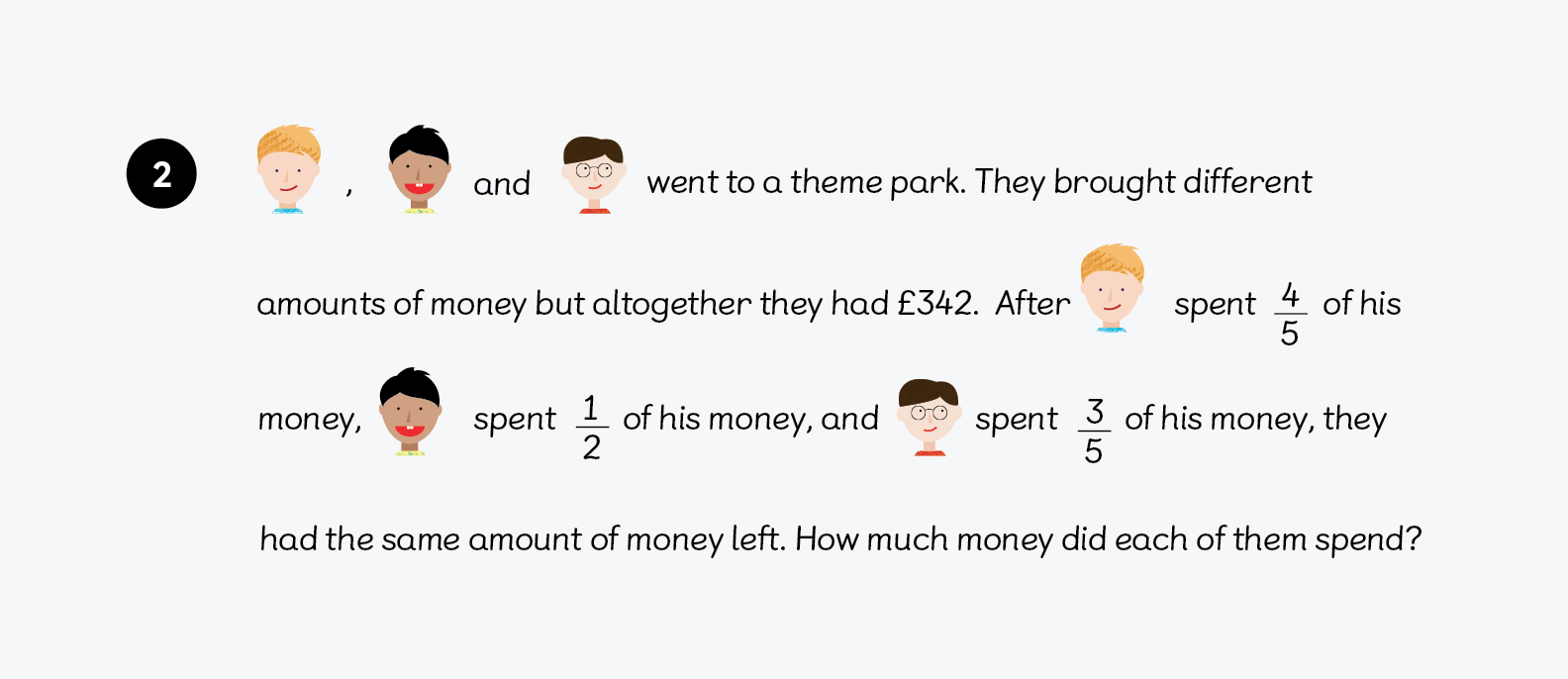
The problem

So, what are the exact steps?

Step 1: Identify and represent the information using bar models

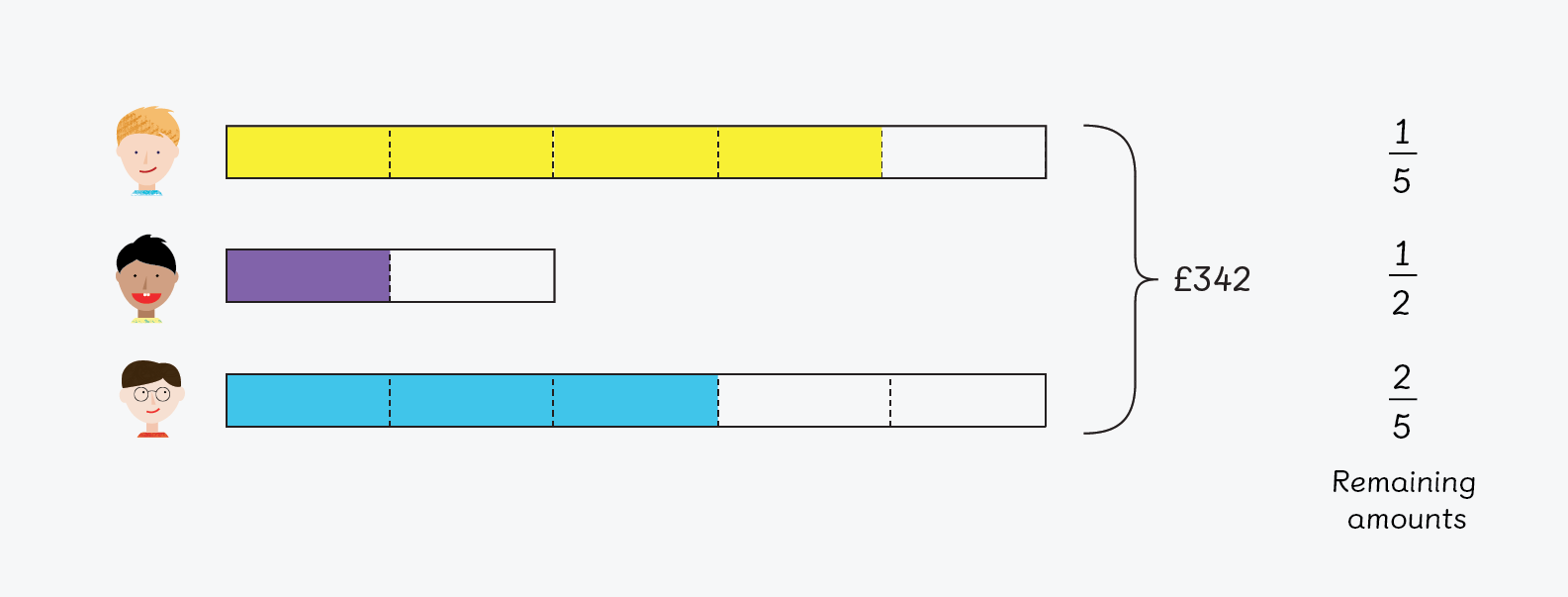
“Draw the 3 bars of the bar model and write the fraction of each remaining part.
This brings you to ¹⁄₅ for Sam, ¹⁄₂ for Ravi and ²⁄₅ for Charles.”

Step 2: Manipulate the information

Previous lessons involved making the bars the same size, and this is helpful here. You need to modify the bar models to do this.

“The question tells you they all had the same amount left. You have to remember that each amount is a fraction of its own amount, instead of a general amount. Using that information, you then change all the numerators into a common number, which here is 2.”

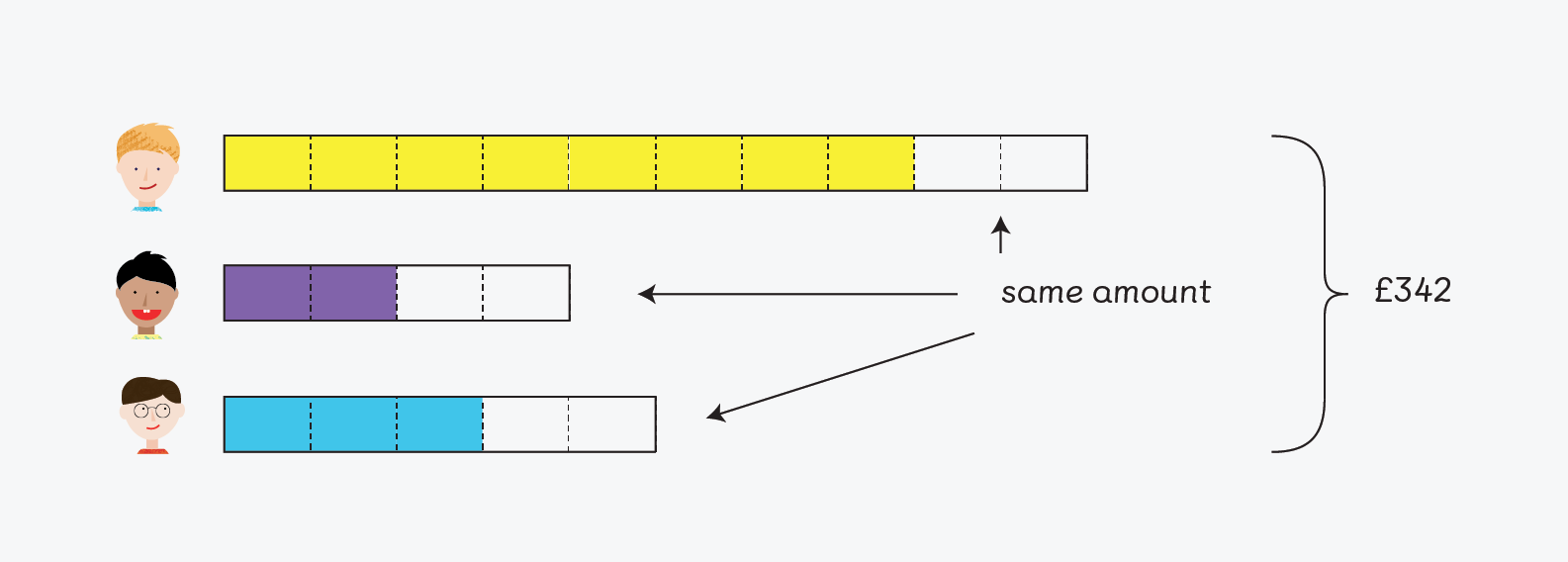
“Do not change the denominators into a common number! If you did, you would be forgetting that each fraction is of a separate whole.”

Therefore,

Step 3: Decide which operations to use

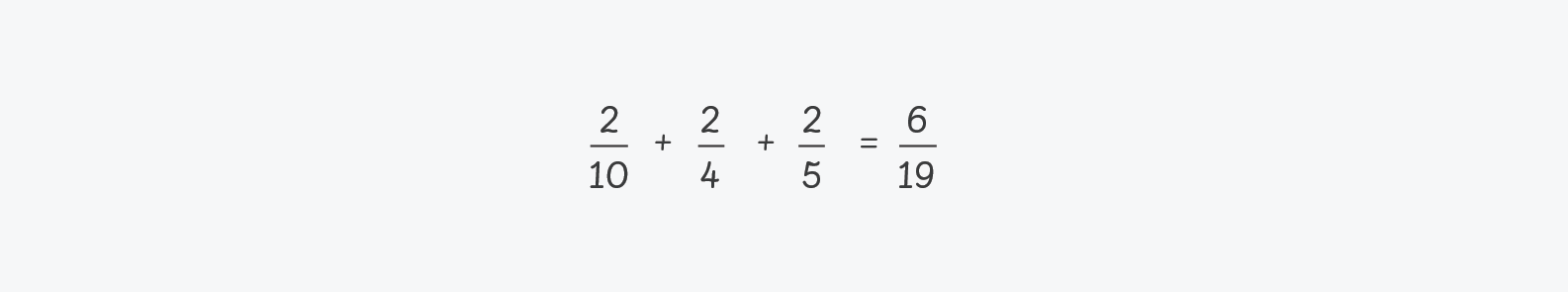
Although it may seem strange to add numerators without first finding a common denominator, it may be helpful to keep in mind you aren’t adding fractions in the usual way. Rather, you are adding pieces of the three bar models that represent how much cash each person has left. The numerators need to be the same because each person has the same amount remaining; they don’t have the same amount to start with.

“You now have a total of 19 pieces of bar (and we know that each person had the same amount leftover at the end, 2 pieces of bar each, or 6 pieces altogether). The total is £342. If we share the £342 by 19, we will find out the value of one piece of bar.”

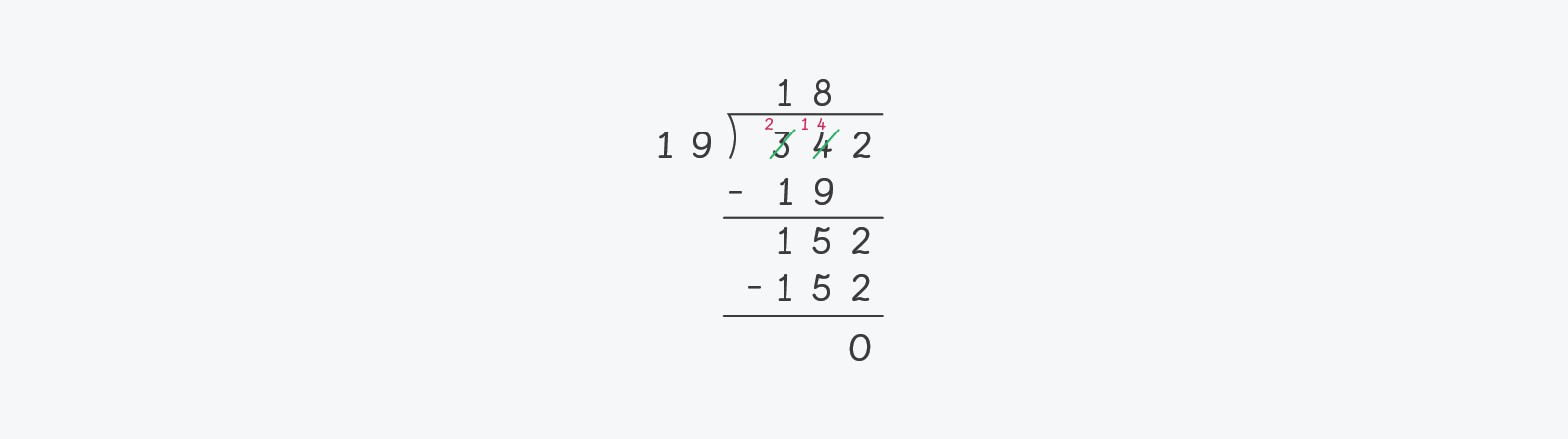
“I divide £342 by 19 using long division. The answer is £18. Therefore, 1 piece of bar is worth £18. Sam, Ravi and Charles each had £36 left from the theme park — two pieces of my changed bar model.

Therefore:
Sam spent 8 pieces of his bar, or £18 × 8.
Ravi spent 2 pieces of his bar, or £18 × 2.
Charles spent 3 pieces of his bar, so £18 × 3.”

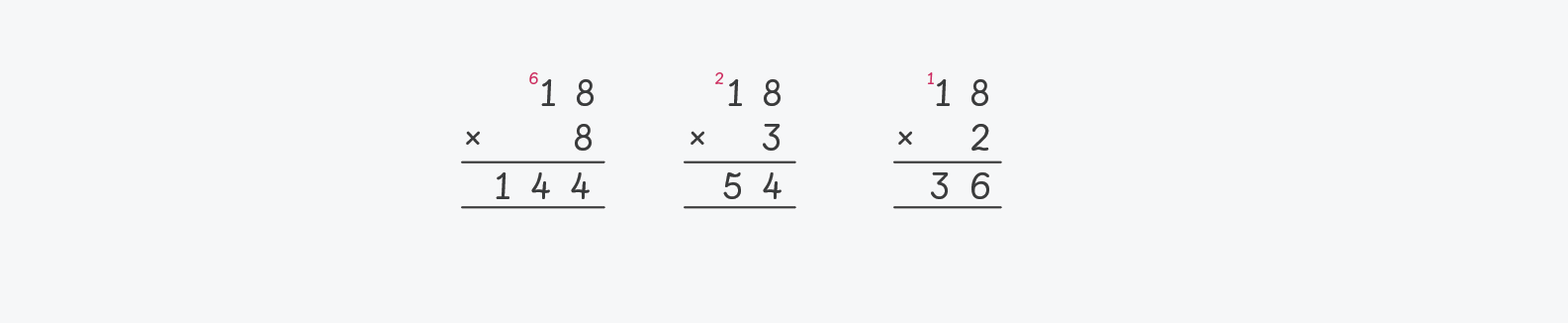
Step 4: Check the answers

“If I worked it out correctly, everything should add up to £342. To check this, I can add what they spent to what they had left, or £36 each.

Here’s my completed bar with workings added to check the answer.”

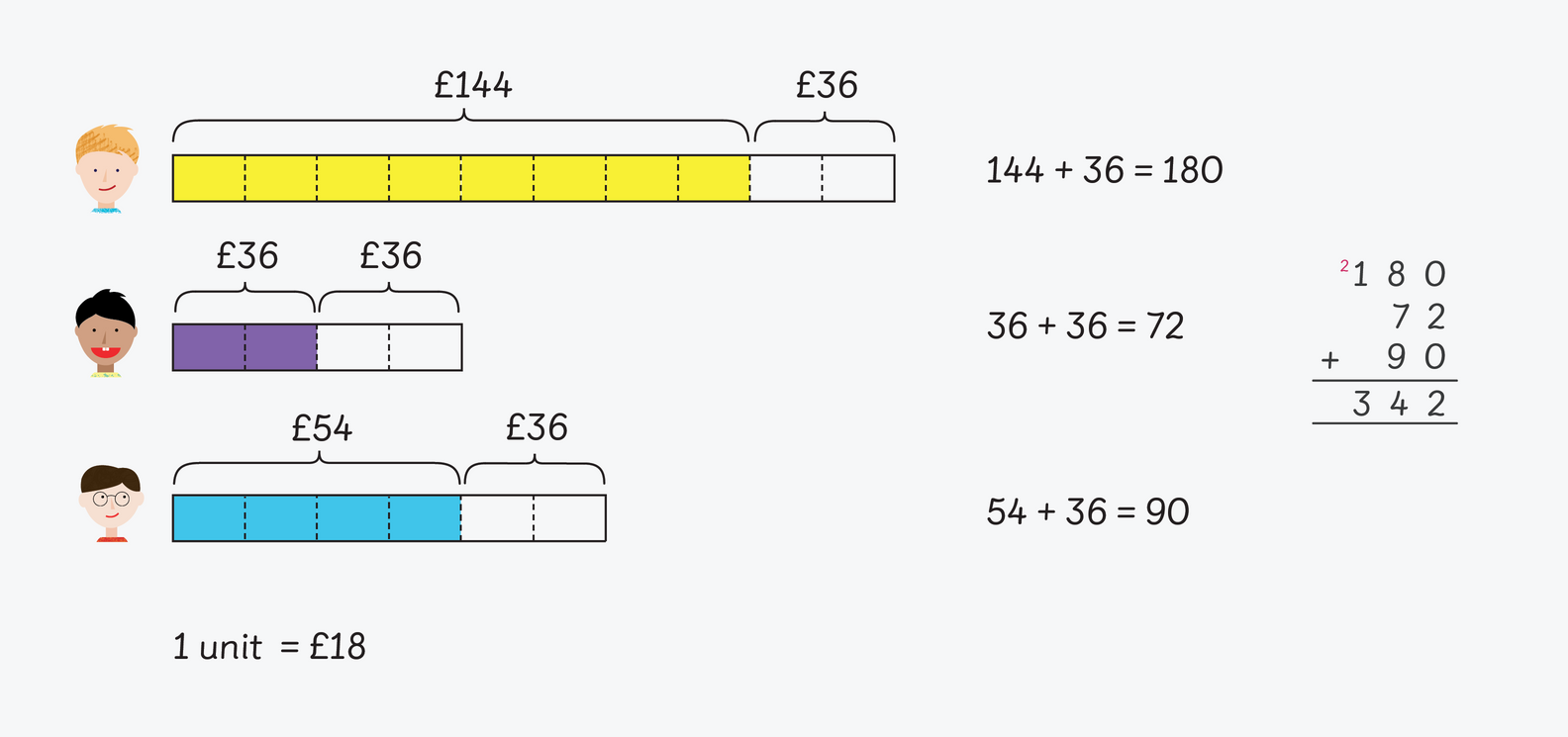
“Sam had £144 + £36 = £180
Ravi had £36 + £36 = £72
Charles had £54 + £36 = £90

£180 + £72 + £90 = £342

The answer is correct — it all adds up to the £342 we started with.

They spent a lot of money at the theme park!”

Class mindset

The questions in the workbook follow a broadly similar line of reasoning, so they can be tackled in any order. As a class, we talked about the idea of ‘profitable struggle.’ If you know the task is going to be hard, try and enjoy the challenge — work through different ideas, talk with people, try again, change groups, etc.

Frustration only occurs if the answer is the one thing — the only thing — that has to be achieved. This isn’t the case with these problems, which should arouse pupils’ interest and challenge them. Moreover, problems such as these can help you see who has properly harnessed the power of reasoning.

I started each of these complex word problem lessons with the assumption that the answer was somewhere in the room. And, I’m pleased to say, so it proved.