How to spot advanced learners using the five core competencies

After a Maths — No Problem! training day focusing on differentiation, I returned to school all fired up. I had decided to focus on the core competencies of a mathematician within my own lessons and in the lessons I observed.

The five core competencies — metacognition, visualisation, generalisation, communication and number sense — can be applied to new and varied situations. Learners can use them to investigate complex problems and clearly communicate their ideas.

Let’s go through the checklist of the five core competencies and look more closely at how advanced learners might demonstrate them.

1. Does your learner demonstrate metacognition?

Metacognition teaches learners to think about how they are thinking. Advanced learners will be aware there can be multiple methods of working out a problem and can choose which method they wish to use. They deepen their understanding by observing how their peers solve problems and incorporating these methods into their own toolkit.

For example, during a lesson on fractions, my Year 5 class came up with several methods for how to solve 6 × 3 ²⁄₃. One learner had that lightbulb moment upon seeing a classmate use a bar model to work out the problem. She was able to think critically about how to solve the problem and realised bar modelling can be a useful method in certain instances.

When they learn new things, advanced learners will reflect on how and why their thinking has changed.

2. Has your learner acquired the skill of visualisation?

Visualisation requires learners to ‘show how they know’ at every stage on their way to solving a problem. Advanced learners develop the ability to visualise through working with concrete materials, engaging in discussion and drawing pictures.

I asked a class of Year 2 learners to compare quadrilaterals and triangles. Each pupil had several of each shape in their hands. The advanced learners could sort them without prompting into different groups and then discuss and justify their groupings.

The Concrete-Pictorial-Abstract (CPA) approach will help your class develop advanced visualisation skills. Eventually, your pupils will ‘show how they know’ automatically.

That’s why the earlier you introduce the CPA approach, the sooner your learners will start to build visualisation skills.

3. How well does your learner generalise?

Generalisation challenges learners to dig deeper by finding proof. Advanced learners look for patterns and test them to see if they can be applied to general situations.

This Year 6 pupil applied her knowledge to investigate the sum of the angles in a quadrilateral. After a little more experimentation, she concluded the sum of the angles in every quadrilateral must always be 360°. How much more powerful is her conclusion when she discovered it for herself?

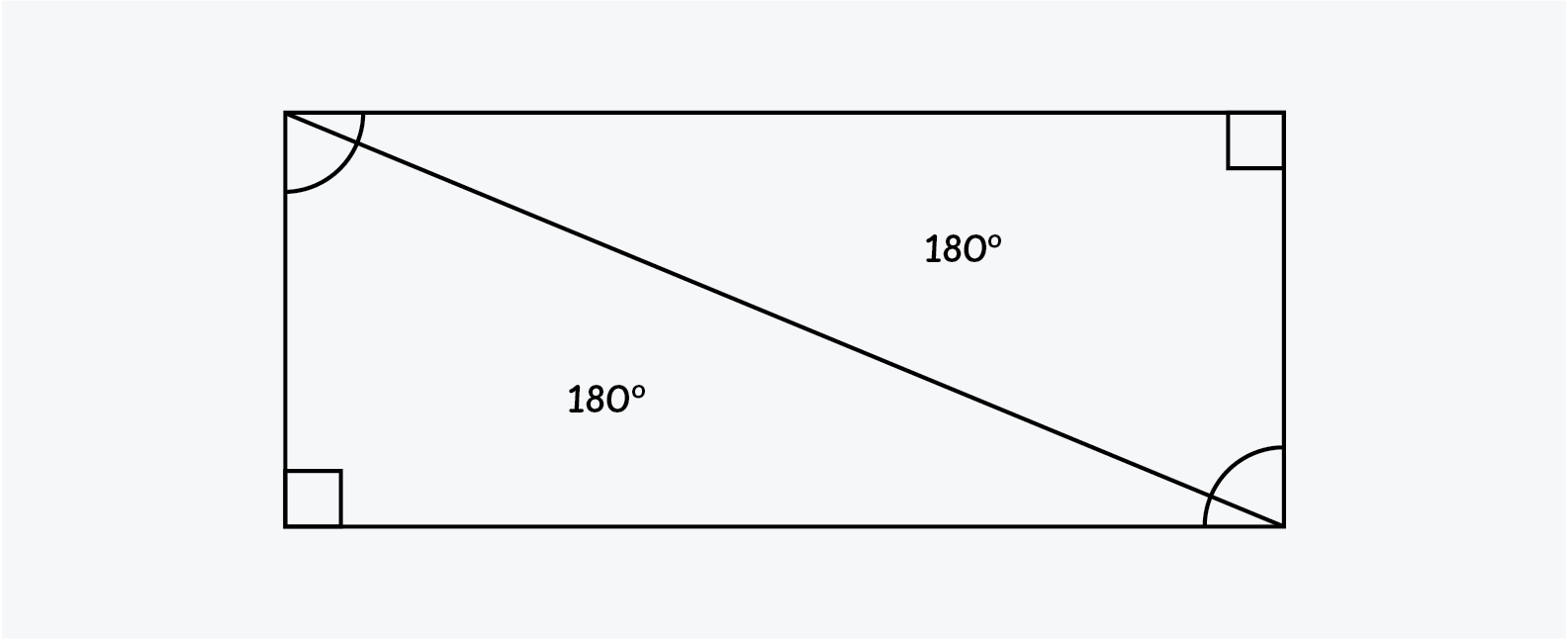
“I drew a rectangle and split it into two triangles. Using previously learnt knowledge, I knew that angles in a triangle add up to 180°, as shown above. Once both triangles add up to 180, we should realise that the angles of this rectangle add up to 360°.”

4. How well does your learner communicate his thought processes?

Advanced learners tend to be able to communicate clearly, and may often answer in full sentences. To encourage clear communication, try asking your pupils to discuss the work they’re doing, or use structured tasks centred around a class discussion. How children communicate their thinking and methods is key to their understanding and development.

In this example, a Year 5 learner used diagrams in his journal to show in three different ways that¹⁄₆ + ¹⁄₃ + ¹⁄₃ = 1.

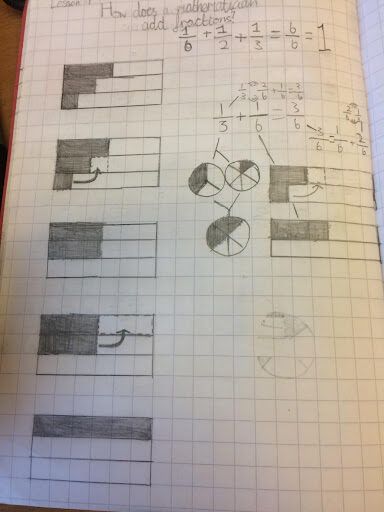
Some children explain their reasoning using words and others use numbers, but advanced learners clearly articulate their thoughts and will structure their journal entries to make them easier for others to follow.

5. Does your learner exhibit good number sense?

Some learners are labelled ‘advanced’ simply because they can accurately recall rules and procedures. For example, a learner who can perform long multiplication accurately every time may be seen by teachers and peers as advanced.

However, these pupils may not be able to articulate the reason for doing what they are doing or apply their knowledge.

If a pupil can perform 56 × 78 using long multiplication, how would she work out 56 × 79? Can she see the connection in the calculations and use the answer to find another? Would she use the same method to calculate 56 × 80? Can she explain how she knows her answer is correct?

Relying on rote learning can give learners a false sense of security. Often, the confidence of these ‘gifted’ learners crumbles once they’re introduced to more difficult material at the secondary or post-secondary level.
That’s why it’s so important to make the distinction between recall and number sense. Delving deeper and thinking flexibly with numbers gives learners the skills and resilience they need to solve unfamiliar problems.

It can be a mammoth task to get learners to not only give the answer but also to explain their methodology and thinking. Slowing down and thinking about ‘why’ is the key to mastery. Developing each facet of the five core competencies will help learners to become well-rounded mathematical thinkers.