Teaching Place Value in Year 1: A Comprehensive Guide for Educators

Place value is a foundational concept in our number system, laying the groundwork for all future mathematical learning. For Year 1 pupils, grasping this concept is a key step in developing a deep understanding of numbers and their relationships.

As educators, it's our responsibility to guide these young minds through the fascinating world of place value, setting them up for success in their mathematical journey.

In this guide, we'll explore effective strategies, engaging activities, and assessment techniques to help you teach place value in Year 1, as well as how parents can get involved at home.

Whether you're a seasoned educator or new to teaching maths, you'll find practical tips and research-based methods to make place value come alive in your classroom.

Understanding place value: Key concepts for Year 1

The UK National Curriculum states that in Year 1, pupils are expected to read, write and count numbers up to 100 using a tens and ones place value through objects and other pictorial representations.

Base-10 number system

Our number system is built on the concept of base-10, which means we use ten digits (0-9) to represent all numbers. In Year 1, we introduce this concept through hands-on activities and visual representations.

Key points to remember:

• Each digit's position determines its value
• The value of a digit increases tenfold as it moves one place to the left
• Zero is an important placeholder in our number system

Try this: Use a place value chart with physical objects to demonstrate how numbers are built. Start with single digits, then progress to two-digit numbers to show how the position changes the value.

Tens and ones

Understanding tens and ones helps pupils visualise numbers and lays the groundwork for addition and subtraction.

Key ideas to emphasise:

• A 'ten' is a group of ten ones
• Two-digit numbers are composed of tens and ones
• The first digit in a two-digit number represents tens, the second represents ones

Try this: Use base-10 materials such as blocks or linking sticks to physically represent numbers. For example, show 34 as 3 sticks and 4 individual blocks.

Developmental progression of place value understanding

Understanding place value is a journey that unfolds gradually in Year 1. Let's explore the key stages of this developmental progression, from foundational skills to more abstract thinking.

Pre-place Value Skills

Before diving into place value, pupils can benefit from certain foundational skills. These pre-place value skills set the stage for deeper understanding.

Key pre-place value skills include:

• One-to-one correspondence: matching objects to numbers
• Subitising: recognising small quantities without counting
• Conservation of numbers: understanding that the number of objects remains the same regardless of arrangement

Try this: Use dot patterns on cards for quick subitising exercises. Start with patterns up to 5, then gradually increase to 10 as pupils become more confident.

Counting and grouping

As pupils progress, they move on to counting and grouping. This supports the understanding of the base-10 system and forms the backbone of place value comprehension.

Focus on these activities:

• Counting objects in twos, fives, and tens
• Grouping objects into tens and ones
• Using ten frames to visualise numbers

Try this: Create a 'counting station' in your classroom with various objects. Encourage pupils to practise counting and grouping during free time, reinforcing these skills through play.

Transitioning from Concrete to Abstract thinking

The journey from concrete understanding to abstract thinking is at the heart of the Maths — No Problem! approach. We teach this through the Concrete-Pictorial-Abstract (CPA) approach.

Stages of the CPA approach:

1. Concrete: Pupils manipulate physical objects
2. Pictorial: They use drawings or images to represent numbers
3. Abstract: They work with numbers and symbols

When introducing a new concept, always start with concrete materials. Gradually introduce pictorial representations alongside the concrete, before moving to abstract symbols. This layered approach ensures pupils build a solid understanding at each stage.

Effective teaching strategies

Teaching place value in Year 1 requires a thoughtful approach that engages young learners and builds a strong foundation for future mathematical understanding. We’ve already discussed the CPA method, but what about other strategies? Let’s find out.

Manipulatives: More than just toys

How we use manipulatives determines how effective they are during our lessons. We can’t just hand out counters and hope for the best. Guide pupils in using these tools purposefully. For instance, when working with place value charts, have pupils physically move objects between columns to demonstrate regrouping.

Try this: create a 'maths toolkit' for each pupil with essential manipulatives like:

• Base-10 blocks
• Place value cards
• Number line
• Ten frames

This ensures everyone has access to these tools when needed, promoting independent exploration and reinforcing place value concepts.

Visual models: Seeing is understanding

Visual models bridge the gap between concrete objects and abstract numbers. Number lines are particularly versatile when teaching relationships between numbers.

Use them to demonstrate:

• Counting forwards and backwards
• Visualising 'one more' and 'one less'
• Comparing and ordering numbers

For a practical activity, create a long number line on the classroom floor. Have pupils physically jump forward for 'one more' and backwards for 'one less'. This kinesthetic approach reinforces the concept while adding an element of fun.

The language of maths

Precise mathematical language is key to understanding place value:

• Tens and ones
• What does the [digit] stand for?
• Place value chart
• Number bonds

Encourage pupils to use this language when explaining their thinking. This not only reinforces their understanding but also develops their mathematical communication skills.

A helpful strategy is to create a 'maths word wall' in your classroom. Add new terms as you introduce them, and refer to the wall regularly during lessons. This visual reference helps pupils internalise the language of place value.

The goal isn't just for pupils to calculate correctly, but to truly understand the underlying concepts of place value.

Engaging activities for teaching place value

We can make teaching maths fun with these hands-on experiences and real-world connections.

Hands-on games and exercises

Try these hands-on activities:

• Place value bean bag toss: Set up buckets labelled 'Tens' and 'Ones'. Pupils throw beanbags and record the two-digit number they create.
• Number building dice: In pairs, pupils roll dice to generate digits, then use base-10 blocks to build the largest number possible.
• Swap shop: Give pupils a pair of digit number cards. They must trade with classmates to make the largest or smallest number possible.

Digital tools and interactive activities

Incorporating technology can enhance place value lessons and cater to different learning styles.

Effective digital resources include:

• Interactive number lines and hundred squares
• Place value games on educational websites
• Virtual manipulatives that mimic physical base-10 blocks

Digital tools should complement, not replace, hands-on learning. Use them to reinforce concepts and provide additional practice.

Real-world applications and problem-solving

Connecting place value to real-life situations helps pupils understand its relevance and importance.

Consider these real-world activities:

• Classroom shop: Set up a pretend shop where items cost up to £99. Pupils use play money to make purchases, reinforcing their understanding of tens and ones.
• Number treasure hunt: Hide two-digit numbers around the school or playground. Pupils must find and order them from smallest to largest.
• Daily calendar: Use a monthly calendar to discuss dates, reinforcing concepts like 'one more' and 'one less'.

Try this: Encourage pupils to spot numbers in their environment and discuss their place value. This could be house numbers, price tags, or page numbers in books.

Differentiation techniques

In your classrooms, you'll find a range of abilities when it comes to understanding place value. Effective differentiation ensures that all pupils are appropriately challenged and supported. Let's explore some techniques to cater to diverse learning needs.

Supporting struggling learners

Pupils who find place value challenging often need more concrete experiences and targeted support.

Try these strategies:

• Use smaller number ranges: Start with numbers up to 20 before moving toward 100
• Provide additional manipulatives: Make sure struggling learners have regular access to manipulatives like base-10 blocks, number lines, and ten frames
• Use visual aids: Create place value charts with pockets to physically 'build' numbers

Try this: Implement a 'maths buddy' system where struggling learners are paired with more confident peers. This peer support can boost confidence on both sides. Pupils who understand the concept can practise explaining what they learned and struggling learners get a different perspective from another student.

Challenging advanced learners

For pupils who grasp place value quickly, provide opportunities to deepen their understanding and apply their knowledge in new contexts.

Consider these extension activities:

• Introduce three-digit numbers: Challenge advanced learners to explore hundreds, tens, and ones
• Encourage problem creation: Ask pupils to create their own place value puzzles for other students to solve

Addressing common misconceptions

Identifying and addressing misconceptions early is important for building a solid understanding of place value.

Common misconceptions include:

• Confusion between place and face value: Some pupils might think the '2' in 24 means 2, not 20
• Difficulty with zero as a placeholder: Pupils might struggle to understand why 105 is larger than 15
• Reversing digits: Writing 24 as 42, for example

Addressing misconceptions:

• Use concrete materials to physically represent numbers, emphasising the difference between tens and ones
• Provide plenty of practice with numbers including zero
• Use place value cards that can be physically arranged and rearranged

Try this: Create a 'misconception station' in your classroom. Display common errors and invite pupils to spot and correct them. This not only addresses misconceptions but also develops critical thinking skills.

By implementing these differentiation techniques, pupils, regardless of their starting point, can develop a robust understanding of place value. The goal is for every child to feel successful and engaged in their learning journey.

Parent involvement

Engaging parents in their child's mathematical learning can significantly enhance understanding of all maths concepts including place value.

Let's explore some effective strategies for involving parents in place value learning.

At-home activities to reinforce place value concepts

Encourage parents to incorporate place value exercises into everyday life. These activities should be fun, simple, and require minimal resources.

Suggested activities for parents:

• Number hunt: During walks or shopping trips, spot two-digit numbers and discuss their place value
• Dice games: Roll two dice to create two-digit numbers, then compare them. Talk about their place value and discuss why they are tens or ones
• Sorting coins: Use 1p and 10p coins to represent ones and tens, building different numbers

Try this: Create a 'Maths at Home' kit for each pupil. Include items like dice, base-10 blocks, and a simple place value chart. This ensures that pupils have access to basic resources for at-home practice.

Communication strategies for parents

Clear, regular communication with parents is key to maintaining their involvement and understanding of place value concepts.

Effective communication strategies include:

• Weekly newsletters: Share what maths concepts are being taught and suggest related home activities
• Parents evenings: Demonstrate how place value is taught in class during your next parent evening so parents can experience the learning process and model it at home
• Online resources: Create a class blog or use a learning platform to share videos of place value explanations and activities

Remember, many parents may be unfamiliar with current teaching methods, especially if they learned maths differently. Be patient and provide clear explanations of your classroom’s maths approach and the importance of place value.

Addressing parental concerns

Parents might express concerns or confusion about place value teaching methods. Address these proactively to maintain their support and involvement.

Common concerns and responses:

• "Why use base-10 blocks instead of just writing numbers?": Explain the CPA approach and how concrete understanding leads to better abstract thinking
• "This looks different from how I learned maths": Acknowledge this, but emphasise how these methods develop deeper understanding and problem-solving skills
• "My child still counts on their fingers": Reassure parents that this is a normal stage of development, while gradually introducing more efficient strategies

Together we can create a supportive environment that extends beyond the classroom and opens up doors for communication to set up pupils for future maths success.

Assessment strategies

You may be wondering, how do we actually know if we are on the right track with our students with all of these strategies. This is where assessment comes into play.

Formative assessment techniques

Formative assessment provides real-time insights into pupils' learning, allowing us to adjust our teaching accordingly.

Try these formative assessment techniques:

• Observation: Watch pupils as they work with manipulatives or solve problems, noting their strategies and misconceptions
• Ask open ended questions: Learning to ask open ended questions leads to a wider understanding of the pupil’s capabilities
• White board responses: Pose quick questions for pupils to answer on individual white boards, allowing for a quick scan of class understanding

Using exit tickets and quick checks

Exit tickets and quick checks provide a snapshot of understanding at the end of a lesson or learning sequence.

Effective exit ticket ideas:

• Demonstrate place value: Ask pupils to represent a two-digit number using a place value chart, base-10 blocks, or number line
• True or false: Provide a statement about place value for pupils to evaluate
• Fill in the blank: Give pupils a partially completed place value statement to complete

Exit tickets should be quick to complete and easy to assess. Use the results to inform your planning for the next lesson.

Tracking progress over time

Monitoring progress over time helps ensure all pupils are moving forward in their place value understanding.

Consider these tracking methods:

• Place value checklist: Create a list of key skills (e.g., can count in tens, can identify tens and ones in a two-digit number) and regularly update it for each pupil
• Regular journalling: Have pupils consistently journal about their place value work throughout the year to show progression
• Regular low-stakes quizzes: Use short, focused assessments to track understanding of specific place value concepts

Using assessment data

The true value of assessment lies in how we use the data to inform our teaching.

Ways to use assessment data:

• Grouping: Use assessment results to create flexible groups for targeted support or extension
• Lesson planning: Adjust your plans based on common misconceptions or gaps identified in assessments
• Individual support: Use one-to-one conferencing to address specific difficulties highlighted by assessments

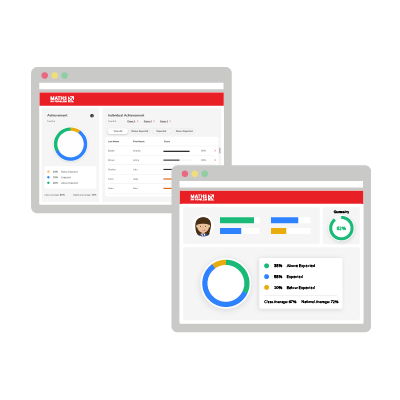
The goal with assessment is to gain a clear picture of each pupil's place value understanding, enabling educators to provide the right support at the right time.

Empowering young mathematicians: Their place value journey begins here

Teaching place value in Year 1 is a crucial foundation for mathematical learning, requiring a thoughtful blend of concrete, pictorial, and abstract approaches to help pupils understand the base-10 number system.

Effective strategies include using manipulatives, incorporating visual models, and engaging in real-world activities, whilst differentiating instruction to support all learners and involving parents in the learning process.

By implementing these varied techniques and maintaining ongoing assessment, we can create a rich, engaging environment for our pupils to develop a robust understanding of place value, setting them up for future mathematical success.