Manipulatives in Maths — A Classroom Guide for Teachers
Mathematical manipulatives are touted as essential tools for learning, but let's be honest—we've all experienced that moment of dread when we hand them out. Suddenly, your carefully planned lesson turns into chaos: One pupil starts building a fortress with the base ten blocks while another hides all the shiny counters.
Yet, despite these challenges, manipulatives play an important role in mathematics education. They bridge the gap between abstract concepts and tangible understanding, helping pupils grasp basic number sense. In fact, the National Curriculum emphasises their importance across all key stages, recognising that hands-on learning is vital for developing maths fluency, reasoning, and problem-solving skills.
So, how can we take advantage of these tools without losing control of the classroom? Let's explore the world of maths manipulatives—what they are, why they matter, and how to use them effectively in your primary school lessons.
What are manipulatives?
It can sound complicated, but manipulatives are simply hands-on tools that make abstract mathematical concepts concrete and visual. They're the building blocks, quite literally in some cases, that help pupils wrap their heads around tricky number ideas through good old-fashioned play, exploration, and modelling.
These learning aids come in all shapes and sizes, from the humble counter to the more elaborate Cuisenaire rods. Their key purpose? To give pupils something tangible to manipulate as they grapple with mathematical concepts. Whether it's using multilink cubes to understand place value or fraction circles to visualise parts of a whole, manipulatives help bridge the gap between 'maths on paper' and 'maths in real life'.
Common manipulatives you'll find in primary classrooms include:
- Multilink cubes
- Numicon
- Counters
- Cuisenaire rods
- Base ten blocks
- Bead strings
- Balance scales
- Dice
- Dominoes
- Clock faces
- Digit cards
- Hundred squares

These tools align perfectly with the National Mathematics Curriculum's aims of developing mathematical fluency, reasoning, and problem-solving skills. By allowing pupils to physically interact with mathematical ideas, manipulatives help build a strong foundation for more complex concepts down the line. They're not just toys or distractions—they're powerful learning tools that can transform how your pupils understand and engage with maths.
Why are they important?
Over the past two decades, research has consistently shown the positive impact of using manipulatives in the classroom. A 2013 report published in the Journal of Educational Psychology identified "statistically significant results" when teachers used manipulatives compared with when they only used abstract maths symbols. This highlights the role that manipulatives play in supporting conceptual understanding and facilitating the progression from concrete to abstract thinking.
Alignment with the CPA approach
The NCETM agrees that physical manipulatives should play a central role in maths teaching. "Manipulatives are not just for young pupils, and also not just for those who can't understand something. They can always be of help to build or deepen understanding of a mathematical concept."
This approach aligns perfectly with the concrete-pictorial-abstract (CPA) progression. Once children are confident using manipulatives or 'concrete' resources, they can then move onto pictorial representations or the 'seeing' stage. Here, visual representations of concrete objects are used to model problems. This stage encourages children to make a mental connection between the physical object they just handled and the abstract pictures, diagrams or models that represent the objects from the maths problem.
Enhance problem-solving
But manipulatives do more than just support understanding — they're powerful tools for enhancing problem-solving skills. By allowing pupils to physically manipulate and visualise mathematical concepts, they can more easily devise strategies to tackle complex problems. This hands-on approach often leads to those 'aha!' moments we all love to see in our classrooms.
Support engagement
Moreover, manipulatives play an important role in fostering engagement and motivation. Let's face it — maths can sometimes seem dry and abstract to young learners. But introduce some colourful counters or interlocking cubes, and suddenly you've got a room full of eager mathematicians. This increased engagement is key to developing a positive attitude towards maths, which in turn supports long-term learning.
This deep understanding allows pupils to move beyond mere memorisation of facts and procedures, towards true mathematical fluency — where they can apply their knowledge flexibly and efficiently across a range of contexts.
In essence, manipulatives are not just helpful additions to our maths teaching toolkit — they're essential components in building a comprehensive, engaging, and effective mathematics education.
Transform Your Maths Assessment
Insights — our online assessment tool — gives you instant, powerful data to identify gaps and improve results.

Types of manipulatives in primary mathematics
In this section, we'll break it common types of manipulatives into bite-sized pieces, just like we do for our pupils.
Physical manipulatives: the classics
These are the tangible, grab-them-with-your-hands resources that have been the backbone of maths classrooms for years. They're the ones that inevitably end up stuck between classroom seats and occasionally in someone's shoe.
Below is a list of common physical manipulatives in the classroom:
Base ten blocks
Ideal for teaching place value, addition, and subtraction with regrouping.
Fraction tiles
Excellent for comparing fractions and understanding equivalence.
Geoboards
Great for exploring 2D shapes, symmetry, and area.
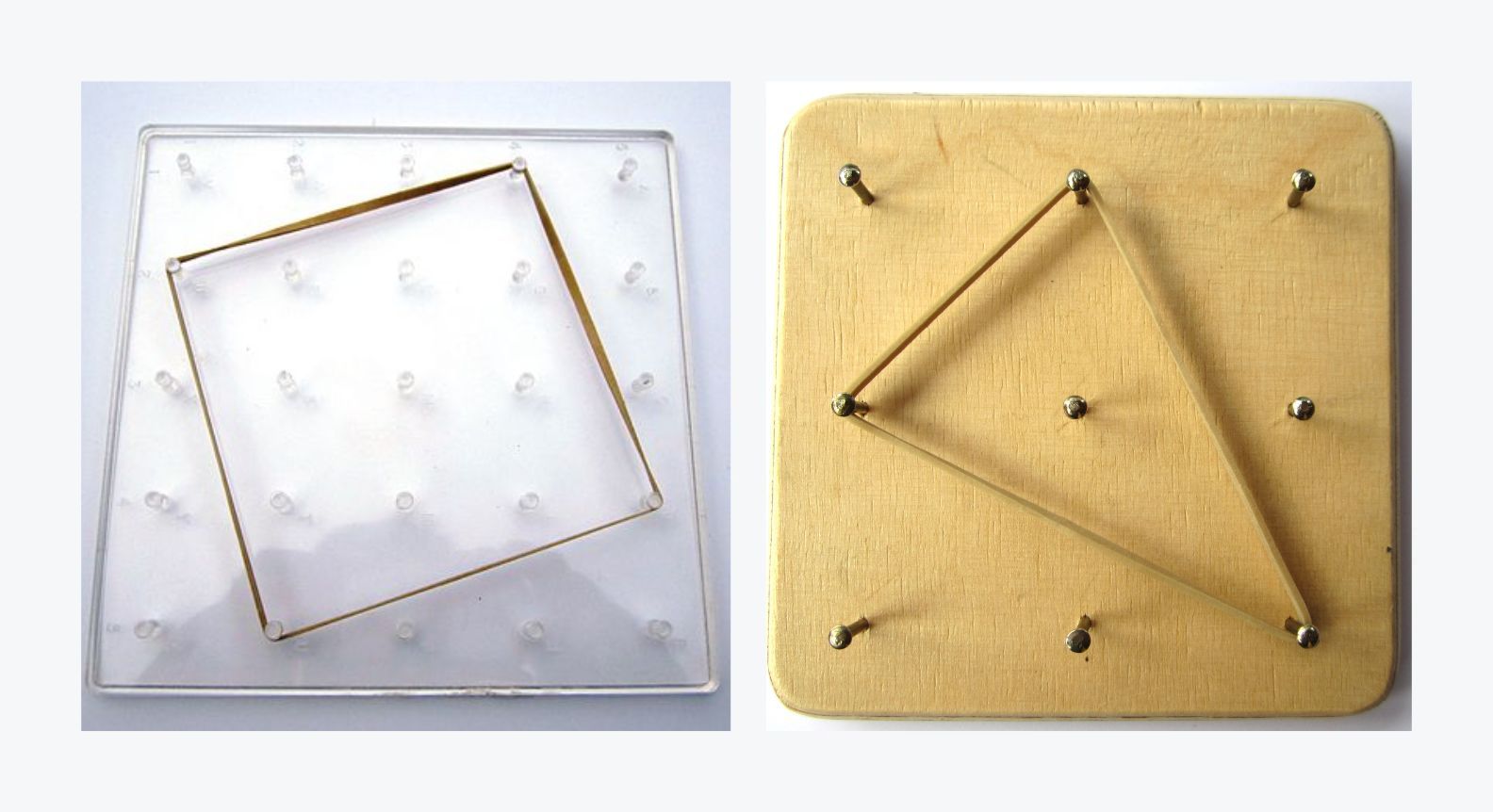
Images: Wikipedia.org
Multilink cubes
Versatile tools for counting, measuring, and understanding volume.
Numicon
Fantastic for developing number sense and exploring number relationships.
Counters
Essential for basic counting, sorting, and introducing simple addition and subtraction.
Cuisenaire rods
Useful for teaching multiplication, division, and fractions.

Image: Pinterest
Bead strings
Helpful for developing number sense and practising skip counting.
Dice
Useful for probability exercises and generating random numbers for various activities.
Dominoes
Great for pattern recognition, matching, and basic addition facts.
Clock faces
Essential for teaching time-telling and understanding intervals.

Digit cards
Useful for place value activities and forming large numbers.
Hundred squares
Excellent for identifying number patterns and supporting multiplication and division.
Virtual manipulatives: a new kind of tool
Manipulatives have gone digital! These are interactive, online versions of our physical favourites. Think of them as the maths equivalent of e-books.
Some popular virtual manipulatives include:
Online number lines
These number lines are zoomable, clickable, and free of the uneven lines that are often the result of our hand-drawn versions.
Digital base ten blocks
All the functionality without the risk of losing pieces under desks.
Interactive fraction tools
Slice and dice up pieces in any way imaginable.
Whether physical or virtual, the best manipulative is the one that helps your pupils understand the concept at hand. Whether that's a handful of multilink cubes or a fancy online simulator, if it's making those mathematical lightbulbs flicker on, you're on the right track!
Implementing manipulatives in the classroom — let them play!
Whether you have a bumper pack of manipulatives, a shared bank of resources or your very own DIY versions, it's important to teach children how to use them independently. Here are some best practices for integrating manipulatives effectively into your lessons:
- Introduce gradually: Bring in manipulatives one at a time. If you don't have enough for each child, set up a 'maths table' where pupils can take turns exploring. This works particularly well with younger years where 'choosing tables' are common.
- Allow for exploration: Give children a chance to play with and explore the manipulatives before using them for instruction. Through this exploration, they can start to imagine how the resource might be useful.
- Guided discovery: Ask open-ended questions to stimulate thinking:
- How could you use this?
- How might this help you when adding or subtracting?
- Why do you think they're different sizes — what could that represent?
- Model usage: Once children are familiar with a resource, introduce a simple maths problem and ask them to use the manipulatives to solve it. Model the problem-solving process step-by-step, then guide children through it.
- Scaffold learning: Start with highly structured activities, then gradually reduce support as pupils gain confidence. For instance, begin with direct instruction on how to use base ten blocks for place value, then move to guided practice, and finally independent problem-solving.
- Align with national maths curriculum objectives: Ensure that your use of manipulatives directly supports National Mathematics Curriculum goals. Here are a few examples:
- Year 1: Using counters or number lines to support addition and subtraction within 20.
- Year 2: Use fraction tiles to help pupils recognise, find, name, and write fractions of a length, shape, set of objects, or quantity.
- Year 3: Utilising place value charts (physical or digital) so pupils can recognise 3-digit numbers (100s, 10s and 1s).
- Integrate into lesson plans: Don't treat manipulatives as an add-on. Instead, weave them into your lessons as essential tools for understanding. Plan specific points in your lessons where manipulatives will be most beneficial.
- Support diverse learners: Manipulatives can be particularly helpful for English Language Learners (ELLs) and pupils with learning disabilities. They provide a universal language of mathematics that transcends verbal communication barriers.

Images: The Average Teacher
Manipulatives across Key Stages 1 and 2
Next, let's break down more examples of manipulatives in the classroom by Key Stage.
Key Stage 1 (Years 1-2): Laying the foundations
In these early years, it's all about getting hands-on with numbers and shapes.
- Number and Place Value: Introduce counters, number lines, and base ten blocks. Pupils can observe how 10 ones form a 'ten stick', helping them grasp place value concepts.
- Addition and Subtraction: Utilise multilink cubes for hands-on learning. Pupils can physically join or separate cubes to represent addition and subtraction operations.
- Fractions: Fraction tiles can be effective tools for teaching fractions. They provide a visual and tactile representation of concepts like 'half' and 'quarter'.
- Geometry: Employ geoboards for creating 2-D shapes. Pupils can then be asked to match these shapes on a 3-D surface to enhance spatial understanding.
Key Stage 2 (Years 3-6): Progressing with Purpose
As our mathematicians-in-training grow, so does the sophistication of our manipulatives. We're not ditching the basics, just building on them.
- Multiplication and Division: Array cards and Cuisenaire rods are useful for these operations. For multiplying by 6, pupils can line up 6 rods of 4 to visualise the concept.
- Fractions, Decimals, and Percentages: Fraction circles can be used alongside decimal place value charts. The 100 square is effective for teaching percentages.
- Geometry: The geoboard is a helpful tool for teaching perimeter, area, and symmetry concepts in a hands-on manner.
- Statistics: Data can be represented using multilink cube bar charts or human pictograms, making statistics more engaging for pupils.
CPA Journey: From Concrete to Pictorial to Abstract
Remember, our end goal is for pupils to solve problems without relying on physical props. Here's how we might progress:
- Concrete: Pupils physically manipulate objects to solve a problem. For example, using counters to work out 5 + 3.
- Pictorial: They draw a picture or diagram to represent the problem. Our 5 + 3 might become five circles and three circles.
- Abstract: Finally, they use mathematical symbols and numbers alone. "5 + 3 = 8."
The beauty of this approach? Pupils can always 'go back' a stage if they're struggling with a new concept. Stuck on an abstract problem? Draw a picture! Need more practice? Grab those counters!
Remember, every child's journey through these stages is unique. Some might race through, others might linger longer at certain points. The key is to ensure they have a solid understanding at each stage before moving on.
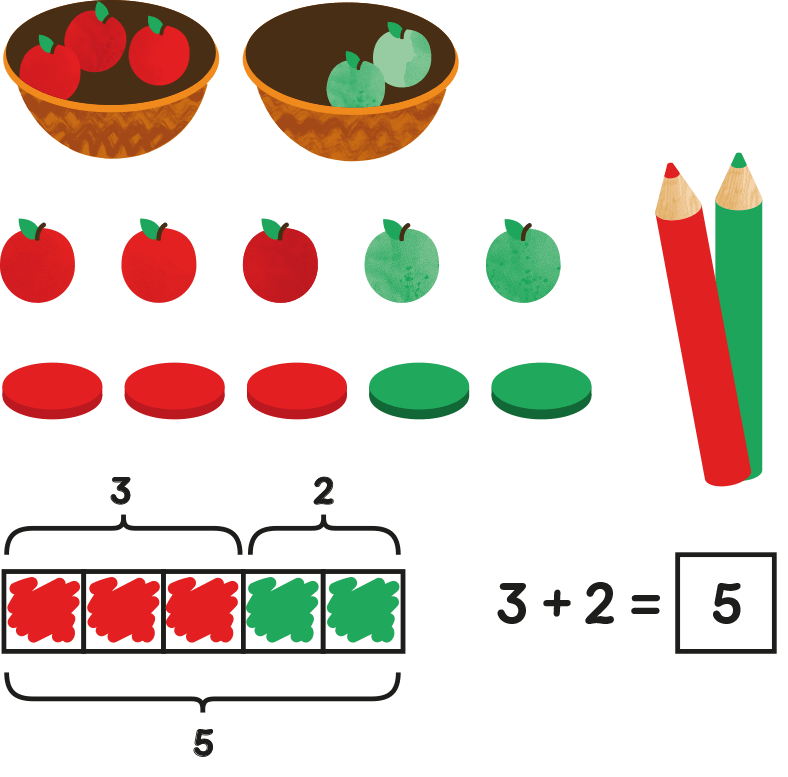
An example of moving from the concrete, to pictorial, to abstract stages.
Manipulative manners
Once you have introduced your resources, speak as a class and explain that they should come up with a set of rules for how they are treated and used. Giving children ownership over the manipulatives as well as the respect to make their own rules will make them feel accountable and lessen the likelihood of negative behaviours when using manipulatives. Write the rules up as a class and display them so they can be referred to.
Storing manipulatives
NRICH recommends children having access to manipulatives “Give open access to all the resources and allow the children free reign in choosing what to use to model any problem they may be tackling. I would make sure that children of all ages had this access from 3 to 11 years old and beyond.” While this is exactly what teachers would like to replicate in their classrooms, not all classes learn in the same way and this isn’t always achievable due to space, budgets, and children’s prior experiences of manipulatives.
Once you have introduced a manipulative, decide as a class where you should store it. You know what works best for your class, so consider different options such as communal drawers, a maths table, individual packs, or a collection of manipulatives for each table. Set clear rules around using and treating manipulatives to ensure they are not broken or lost. Additionally, you could create a monitor for each resource so the children can take ownership and make sure they stay tidy and accounted for.

Creating a classroom culture that uses manipulatives will aid children’s fluency and help develop their ability to solve problems, reason mathematically, and share! If manipulatives are introduced in a considered and gradual way, with clear boundaries from an early age, children should see them as part of everyday learning and not a novelty. They will be seen as tools instead of toys — and hopefully no more multilink towers!

